[Please watch the video attached at the end of this blog for a visual explanation of this topic]
This article will guide you through the subtopic of “Factorisation” under the main topic of “Algebra”.
This topic of factoring or factorising trinomials is the complete opposite of simplifying algebraic expressions, which we discussed before.
When simplifying algebraic equations, we used distributive law to expand the brackets, looked at common terms and then simplified it. Here however, we do something entirely different.
Example 1:
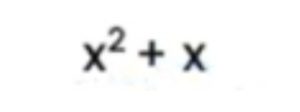
In this example, we can see that both terms (x^2 and x) have one x in common. So we can pull out that x outside the bracket.
The first term is x^2, and once we take out one x from this, another x gets left behind, as x ✕ x = x^2. Since we have already taken the x out of the second term, that just leaves us with 1.
Therefore, the factorisation of this example is this:
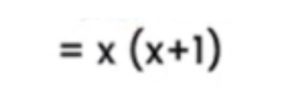
Example 2:
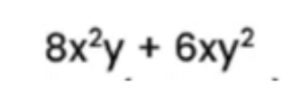
In this, we must first identify the common terms. We can see that one common term is 2, since both 8 and 6 have factors of 2. Another common factor is x, because both terms have at least one x in them. The last common term is y, because just like x, there is at least one y in both terms.
Common terms: 2 ✕ x ✕ y = 2xy
Once 2xy is taken out as the common term, we must see how the first term and and the second term changes accordingly.
Once we take out 2xy from the first term, that leaves us with 4 and x because 4 ✕ 2 = 8, and we need another x to get the x^2.
Similarly, when we consider the second term, we need a 3 there as 3 ✕ 2 = 6, and y ✕ y = y^2.
![]()
- It is very important to factorise very carefully, and extra attention must be paid to the powers involved.
The other type of factorisation is one where you may have to group together the terms and factorise the first two terms and the second two terms separately, and then do an overall factorisation.
This might sound a bit confusing, so for us to easily understand this concept, let’s look at an example.
Example 3:
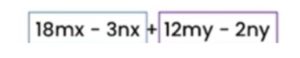
We will first consider the terms highlighted in blue, and then consider the terms highlighted in purple.
In the first two terms highlighted in blue, we can see that 18 and 3 have a common factor of 3, and that there is also an x in both terms.
So we’re going to take out 3x there, which leaves us with 6m –n.
When looking at the next two terms highlighted in purple, 2 and y are common factors. Once 2y is taken out, we are left with 6m – n yet again for the second pair of terms.
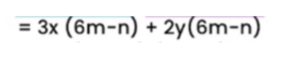
Since the two brackets that we have factored here are the same (6m – n) , we can put that as a separate bracket altogether, and then take off the remaining terms of 3x and 2y to form a separate bracket. So overall, the factorisation comes down to a double bracket factorisation as indicated here:
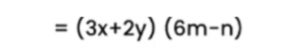
- When it comes to such questions, pay extra attention to your plus signs and minus signs!
Factorising Quadratic Expressions
Example 1:
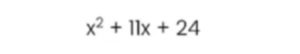
In such a quadratic expression, if there is a value such as x^2, and the number in front of x^2 is one (1), then what we must do to identify and factorise this, is find numbers that multiply to give the last term (24 in this instance), and add up to give the middle term (11x in this instance).
It can be seen that what has been done in step 1 is we have listed down all the factors of 24.
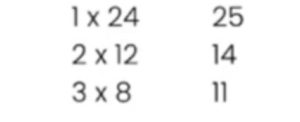
Using these factors, we must see which two factors add up to 11. Only 8 and 3 add up to 11, therefore, those are the numbers we need in this factorisation.
Once we are done with this step, we have x and then you put in the factors that you have, resulting in this:
x^2 + 3x + 8x + 24
We can separate it into two pairs of terms like in example 3.
x (x + 3 ) + 8 (x +3 )
Therefore, the final factorisation of x^2 + 11x + 24 is
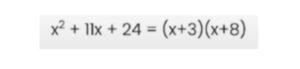
- Be very careful of how and where to include the double brackets.
Quadratic expressions with a twist
When the number in front of x^2 in a quadratic expression is not 1, but a bigger number, we follow the same method set out in example 1 under Factorising Quadratic Expressions. However, before starting with any of those steps, you must multiply the last term and the first term together to know which numbers add up to the middle term.
You can watch the video for a detailed breakdown of such an example. Remember, keep a piece of paper so you can attempt it beforehand!
Master Factoring Trinomials!
Factoring Trinomials and Quadratic equations is closely related to the lessons Factorisation and Indices. To revise these two areas, you can watch the videos here and read the articles on factors and indices as well.
Algebra is an area that students tend to give up on because it looks hard and complicated. In reality, it is one of the simplest concepts to learn. Factoring/ factorising is an area you can score well in one you have got the basics down. Some questions can be found here as well, and you can time your answers to see if you can stick to the time limit given.
If you are struggling with IGCSE revision or the Mathematics subject in particular, you can reach out to us at Tutopiya to join revision sessions or find yourself the right tutor for you.
Watch the video below for a visual explanation of the lesson on mastering factorising trinomials! Don’t forget that there is a timed quiz to test whether you have understood the lesson!
